Generalization of the Simplest
Autonomous Chaotic System
Buncha Munmuangsaena,
Banlue Srisuchinwonga, J.C. Sprottb
aSirindhorn International
Institute of Technology (SIIT), Thammasat University, Pathum-Thani
12000, Thailand
bDepartment of Physics,
University of Wisconsin, Madison, WI 53706, USA
Received 19 October 2010, Received in revised form 7 February 2011,
Accepted 12 February 2011, Available online 16 February 2011
ABSTRACT
An extensive numerical search of jerk systems of the form x''' + x'' + x = f (x')
revealed
many cases with chaotic solutions in addition to the one with f (x')
=
x'2 that has
long been known. Particularly simple is the piecewise-linear case with f (x')
=
α(1− x') for x' > 1 and zero otherwise,
which produces chaos even in the limit of α→∞. The dynamics in this limit can
be calculated exactly, leading to a two-dimensional map. Such a
nonlinearity suggests an elegant electronic circuit implementation
using a single diode.
Ref: B. Munmuangsaen, B. Srisuchinwong, and J.C. Sprott,
Phys. Lett. A 375, 1445-1450 (2011)
The complete paper is available in PDF
format.
Return to Sprott's Books and Publications.
Fig. 1. Attractors of Eq. (3) for each of the nonlinear functions in
Table 1.

Fig. 2. The largest Lyapunov exponent and bifurcation diagram of Eq.
(3) for
f (
x') = −
A exp(
x') with 0 <
A < 0.5.

Fig. 3. Homoclinic orbit in Eq. (3) for
f (
x')
= −
A exp(
x') with
A = 0.1306.

Fig. 4. Attractor for the piecewise-linear system.

Fig. 5. Poincaré section at
x'
= 0 for the piecewise-linear system.

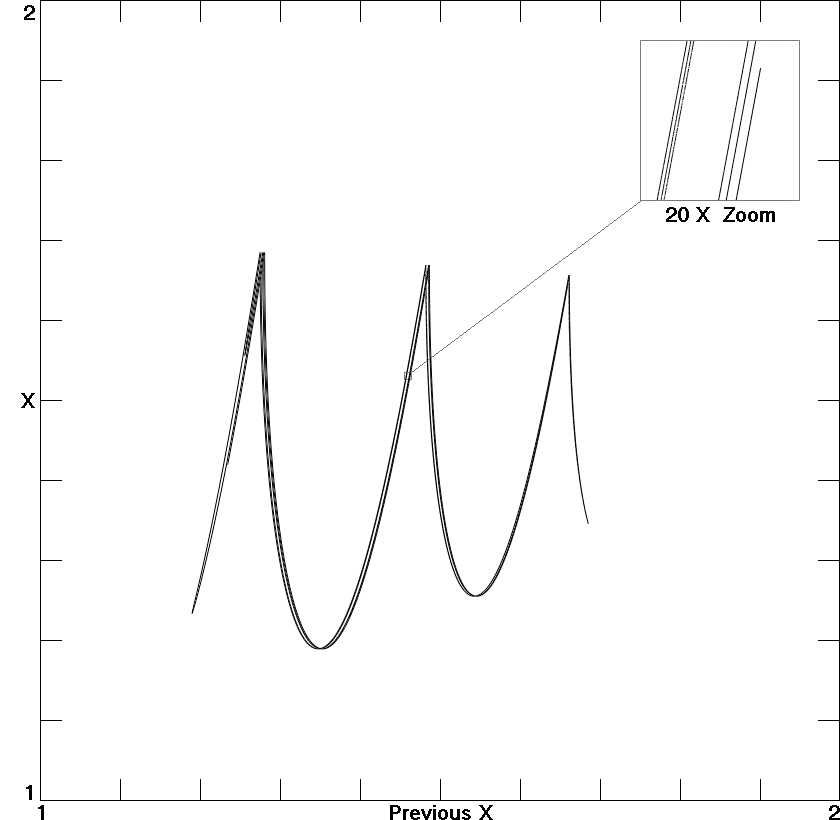
Fig. 6. Return map for the maximum value of
x for the piecewise-linear system.