Fig. 2. Attractor for the maximally complex Henon map with alpha = 1 and b = 0.542 72 along with its basin of attraction.

Fig. 3. Attractor for the maximally complex Lozi map with a = 1.7052 and b = 0.5896 along with its basin of attraction.
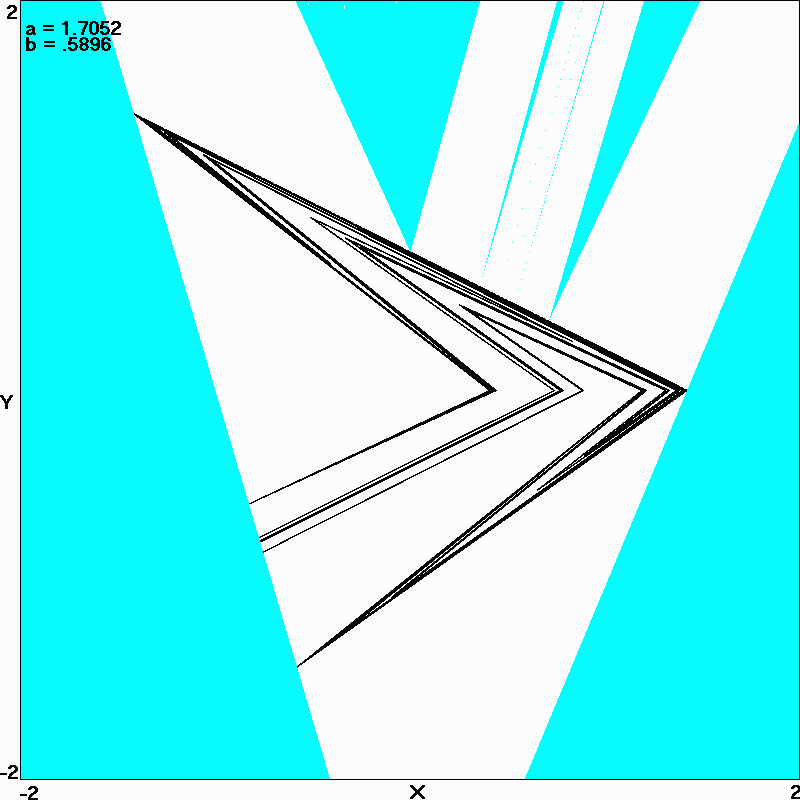
Fig. 4. Attractor for the maximally chaotic normalized Lorenz system with alpha = 0.300, gamma = 0.028, and beta = 0.250.

Fig 5. Attractor for the maximally complex Lorenz system with R = 3.4693.

Fig. 6. Poincaré section at y = 0 for the maximally complex Lorenz system with R = 3.4693.

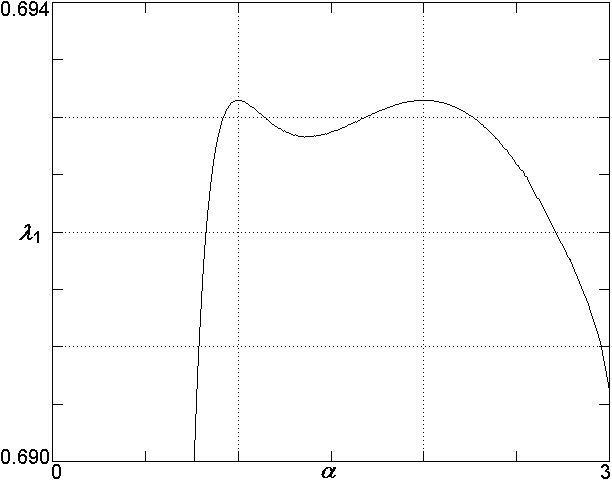
Fig. 7. Variation of the Kaplan-Yorke dimension with the parameter R for the diffusionless Lorenz system in Eq. (8).

Fig. 8. Attractor for the maximally chaotic Rössler system with a = 0.395, b = 0.487, and c = 8.164.
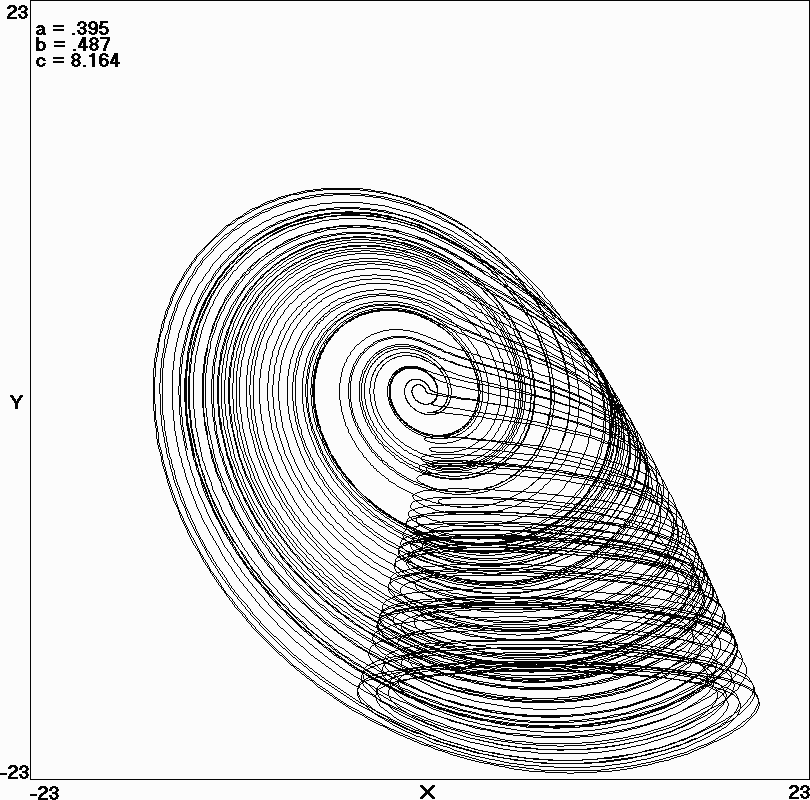
Fig. 9. Attractor for the maximally complex Rössler system with a = 0.6276, b = 0.7980, and c = 2.0104.

Fig. 10. Poincaré section at z = z-* for the maximally complex Rössler system with a = 0.6276, b = 0.7980, and c = 2.0104.
